Consumption-CAPM
Oh, Hyunzi. (email: wisdom302@naver.com)
Korea University, Graduate School of Economics.
Main References
- Kim, Seung Hyun. (2024). "Asset Pricing and Term Structure Models". WORK IN PROGRESS.
Fundamentals of Asset Pricing
Basic Terminologies
Assume the following notations:
- Here,
- Here,
- The goal of asset pricing is to find that the price
- The goal of asset pricing is to find that the price
The rate of return of the asset at time
Using ^a14380Definition 1 (rate of return), we have
- the asset is risk-free: the rate of return of the asset is known at time
- the asset is risky: the rate of return of the asset (
- amount of risk of the asset at
- profitability of the asset at
- an asset is said to be high-risk and high-return if
- amount of risk of the asset at
The risk premium, or expected excess return of an asset at time
Capital Asset Pricing Model
- Many homogeneous investors
- Two-period model: the economy lasts for two period of
- Many risky assets and one risk-free asset: mean and covariance of the vector of risky asset rate of return
- Mean-variance utility: given a portfolio with expected rate of return
Note that a portfolio with weights
- rate of return:
- expected rate of return:
- risk premium:
- variance (risk):
Thus the problem is to find the vector of weights
Thus we have
The optimal portfolio, i.e. the market portfolio has
- rate of return:
- expected rate of return:
- risk premium:
- risk (variance):
From the formula of risk premium of asset
- the idiosyncratic part of risk premium of the asset
- the coefficient from the regression of
- determining how much the factor loads onto the risk premium of asset
- how much
- the
From the formula of risk premium of asset
- the common part of the risk premium of each asset.
- as
Consumption-CAPM
Model and Solution
- Many homogeneous investors.
- Multi-period model: the economy starts at times
- Many risky assets and one risk-free asset:
- there exists one consumption good, whose price normalized as
- there exists
- at time
- at time
- at time
- there exists one consumption good, whose price normalized as
- General utility function: the representative investor has instantaneous utility function
- discount factor is given as
- wage income is given as
- discount factor is given as
The investor's problem is
The Lagrangian is
Thus the Euler equation is
In asset pricing literature, the Euler equation is interpreted as a pricing formula,
From Euler equation, the price of asset
- the discounted marginal utility of future consumption (
- which gets smaller if the investor expects to consume more tomorrow than today.
- which discounts the asset
Beta Representation
From the pricing formula,
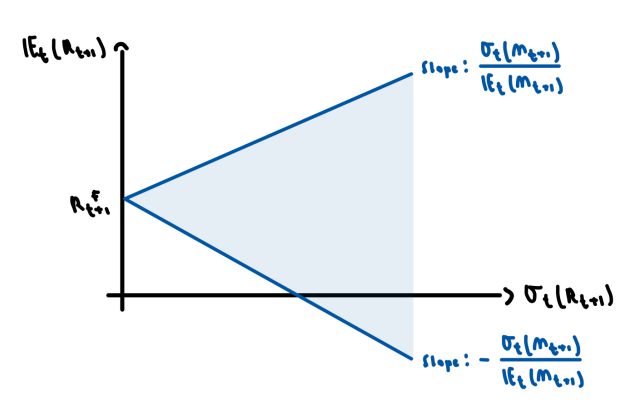
Sharpe Ratio
The Sharp ratio (SR) of an asset is an indicator of the profitability of an asset relative to its risk. The SR of asset
The higher the SR, the grater the the profitability of the asset compared to other assets with the same amount of the risk.
Using the price formula, we can express
Also, note that by the ^fd2d45Definition 8 (sharp ratio), we have
Special Cases of C-CAPM
Case of Log-Normal Returns
Suppose now that the log of the SDF
Note that when the random variable
Similarly, for the risk-free asset
We can also re-define the ^fd2d45Definition 8 (sharp ratio) as
Case of CRRA Utility
From ^187b53Assumption 6 (assumptions for C-CAPM), now assume for the special utility function of CRRA utility:
Then, the ^96b178Definition 7 (stochastic discount factor) is
Defining a consumption growth as
Then, the risk premium of asset